Musical Space

In the physical world, the concept of dimensions—width, length, height—is a way to explain space. We can use the system of Cartesian co-ordinates to “plot” points and objects in space with precision on three axes (right). In music, of almost any style, we can also use an idea of “dimensions” as a kind of metaphor for how “musical space” is organised and perceived.

Unlike in physical (three-dimensional) space, there are only two primary “dimensions” in music.^[1] These are pitch and time.

Of these, the easiest to describe is time: it refers to the duration of a musical sound (or silence) is maintained.  Pitch is a more complex phenomenon and requires further explanation.

Pitch

Listen to the the following sound:

This is the sound of an oboe (left) playing a musical note. Audiences often hear this note before the start of an orchestral concert, as it is the note to which the rest of the orchestra “tunes up.”  This note is conventionally called “A” or, more precisely, “A440.”

Let’s break this down: “A” is the name generally assigned to this note (we will cover note names soon) and “440” refers to the fundamental frequency of this particular “A.”

When our oboist blows this A, a periodic wave form—a regular cycles of compression and rarefaction of air molecules—is established in the column of air inside the body of their instrument, which resonates and amplifies the pattern. These sound waves propagate from the resonating instrument through the surrounding air. When the sound waves reach our ears, our ear drums vibrate and transmit a signal to the brain which we perceive as the oboist’s note.

Most instruments do not product a simple, single wave form.  The oboe is no exception, its A440 (or any other note) is a complex tone comprising many periodic wave forms of different frequencies, called partials.  The fundamental frequency is the slowest (or lowest) of these partials and it is usually also the strongest.  When our oboist blows their A440, there are 440 cycles per second of the fundamental frequency.

The measurement of “cycles per second” is given in units called hertz, abbreviated Hz.  The oboe’s A440 is a note sounding at 440Hz—in other words, its pitch is 440Hz.

Pitch = “height” | TIME = “length”

For a complex set of physiological, cognitive and cultural reasons, most humans (and human societies) attribute a notion of “height” to pitch, with notes whose fundamental frequency is faster being perceived as “higher”—in fact, when we talk about frequency as a number (of cycles in a given period) we talk about “low” and “high” frequency.

To illustrate this idea, play this next sound:

You will likely have heard this as being “higher” than the earlier sound.  It is still an oboe, and it is still playing a note called “A”.  The difference is that the fundamental frequency of this new “A” is 880Hz—exactly twice that of the earlier “A.”  It is a higher note—intuitively, to most people, it will sound “higher” and, “scientifically” speaking, its fundamental frequency is higher.

Thinking back to the beginning of this chapter, we could represent the two oboe As in “musical space” graphically on a two-dimensional plane. Imagine that the two notes are played in succession—each is about five second long.  We could use a simple Cartesian plane to show their duration (length of time) on the x axis and their pitch (“height”) on the y axis  (see Example 1).

When we come to reading and writing music (communicating pitch and time symbolically), this is an inefficient way of representing notes for practical purposes. Even writing a simple and familiar tune such as “Happy Birthday” would be quite cumbersome this way, let alone music of any complexity. In subsequent chapters, we will explain the much more convenient system of Western musical notation which is used to write, read and communicate the essentials of musical pitch and time (as well as other things).  This is known as staff notation.  One basic thing that staff notation shares with the graph in Example 1, however, is the idea that the time relationships of musical notes are shown horizontally and their pitch relationships are shown vertically.

More on frequency and pitch

Let’s look at another representation of our oboe’s two “As”—440 and 880—see Example 2.

In the representation in Example 2, the time scale at the bottom—note the very small time increments in tiny fractions of a second—shows how the cyclic variation in air pressure (a result of the relative density of air particles) is twice as frequent for the higher pitch.  In other words, at A440 there is one cycle of high and low air pressure every 0.00227 seconds, but there are two cycles at A880.

These cyclic patterns can be represented as a curve. For an oboe playing a given note, however, the actual sound is much more complex than this and consists of a number of different frequencies (partials) heard simultaneously above the fundamental frequency.  If we were to strip away all the other frequencies and hear just the fundamental tone, the sound is very different, although the basic pitch remains identifiably the same.

One of the simplest representations of a single frequency, such as the fundamental, is the sine wave, which is a graph of the trigonometric sine function.  It appears as a smooth, regular curve. If we use the signal created by this simple wave form, with a frequency of 440Hz, you will hear the sound as having the same pitch as the oboe A440.  You can play them both here:

You will hear how the sine town sounds much more bland than the oboe and for this reason is often called a “pure tone.”  Nonetheless, the reason we hear the sine tone above and the oboe as having the same pitch is because the oboe’s fundamental frequency is the same as that of the sine wave.

Quantising pitch

Pitch and duration are continuous phenomena. On the pitch dimension, the average human ear can detect sound wave frequencies from about 20Hz to 15,000Hz (15kHz).^[2] On the duration (time) dimension, human cognition tends to interpret events that are separated by less than 100ms as continuous rather than discrete, while events that are separated by much more then 1.5s tend be heard as disconnected, in the sense of not belonging to a single larger phenomenon.^[3]. Within these limits, the values in the dimensions of pitch and duration or timing of musical events each sit on a continuum of value.

Music (with a few exceptions) typically does not operate on these continua. Instead, pitch and duration are quantised.  In this chapter, we will look at how this is commonly done for pitch in Western music. The following chapter will investigate duration.  This will introduce us, also, to the fundamentals of music notation—common ways of writing music in terms of these two dimensions.

In Western music a note with a fundamental frequency of 440Hz is designated “A.” This is only one of several frequencies, however, that are called A.  In standard Western tuning,^[4] every pitch with a fundamental frequency that expresses a simple relationship with 440Hz through repeated doubling or repeated halving is generically called “A” in the Western system.

Why are these notes all called “A”? The answer lies in the very simple mathematical relationships of their fundamental frequencies, which lie on a geometric sequence (1, 2, 4, 8, 16…).  These relationships can be shown graphically by comparing the wavelengths of the fundamental frequencies and by listening to them.  In the graphic below, simple waveforms for the fundamental frequencies of these As are illustrated, and piano notes for these frequencies can be heard by clicking on the numbers on the left. You will hear when you click on these frequencies that there is a strong relationship between them; we perceive that they are alike.

The clear sonic relationship these notes have with one another is called octave equivalence, and this is probably the most fundamental relationship between different musical notes in the Western system (and many others).  Another way of conceptualising this is that these notes belong to a group or class of notes.  In the examples above, this is the pitch-class “A.”

We now have two different ways of conceptualising “A” in music:

1. as any particular given note A (e.g. A440, or A220), or
2. as any one of a class of notes A.

Naming pitch and pitch class: letters A to G

Between any two adjacent notes A,  Western music makes further discrete divisions into intervening notes.  Traditionally there are six additional named pitch classes, following the letters of the alphabet.  This give us seven note names in all: A, B, C, D, E, F and G.  Once we get to G, the pattern returns to A and starts again.  There are two ways to visualise this:

• a line extending infinitely in either direction—higher to the right, lower to the left.
• a circle around which the letters a are arranged—higher going clockwise, lower going counter-clockwise.

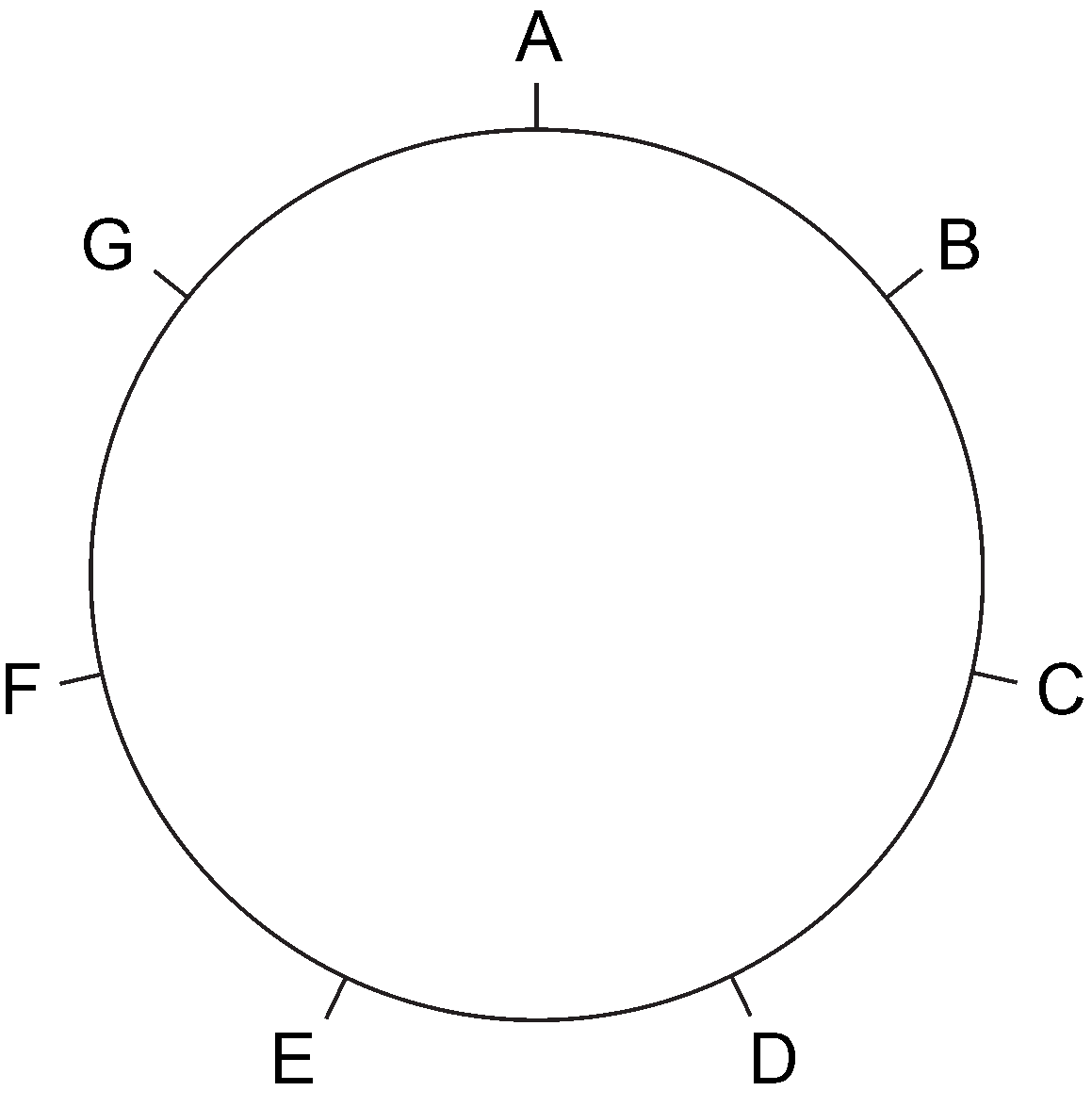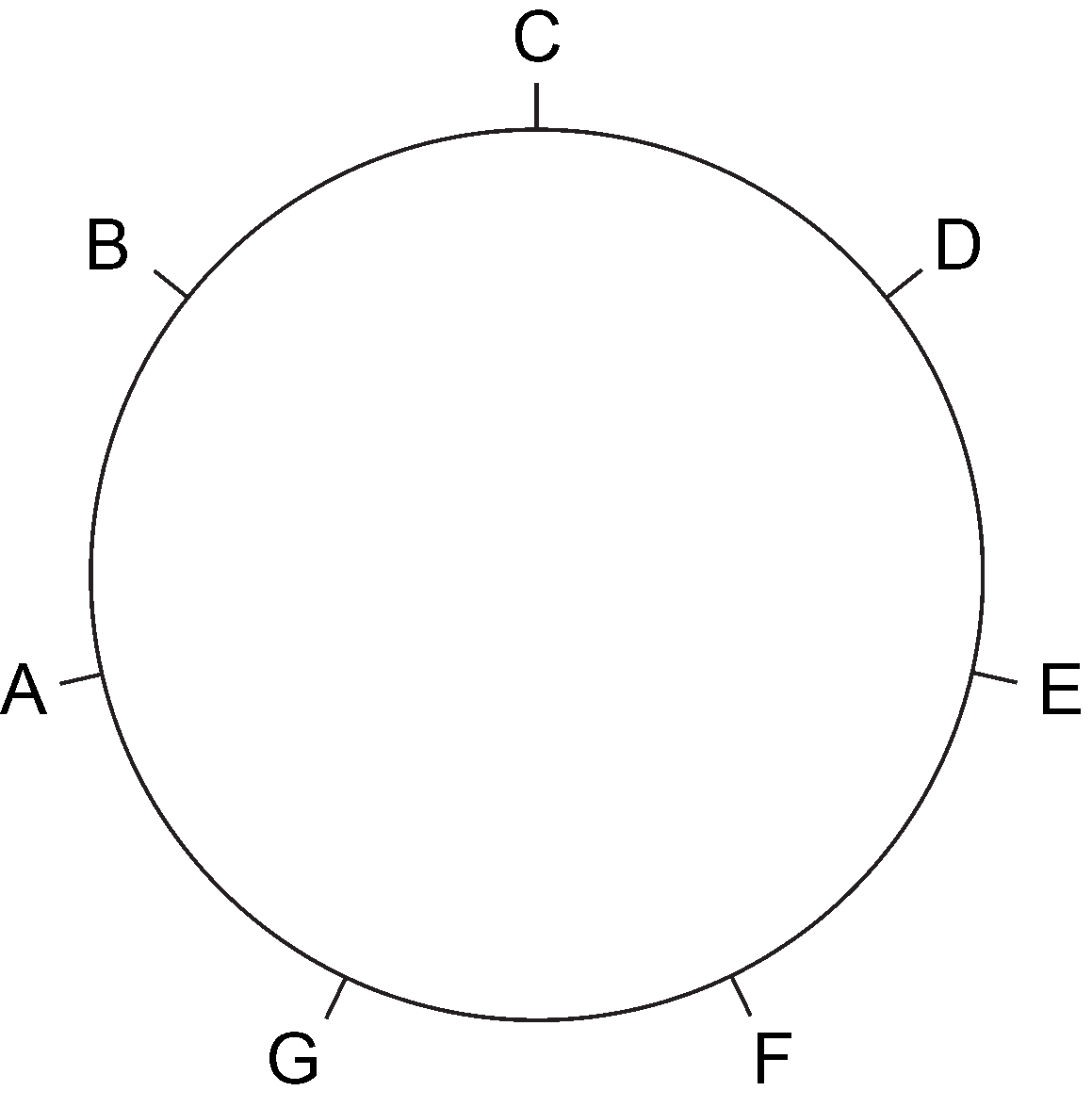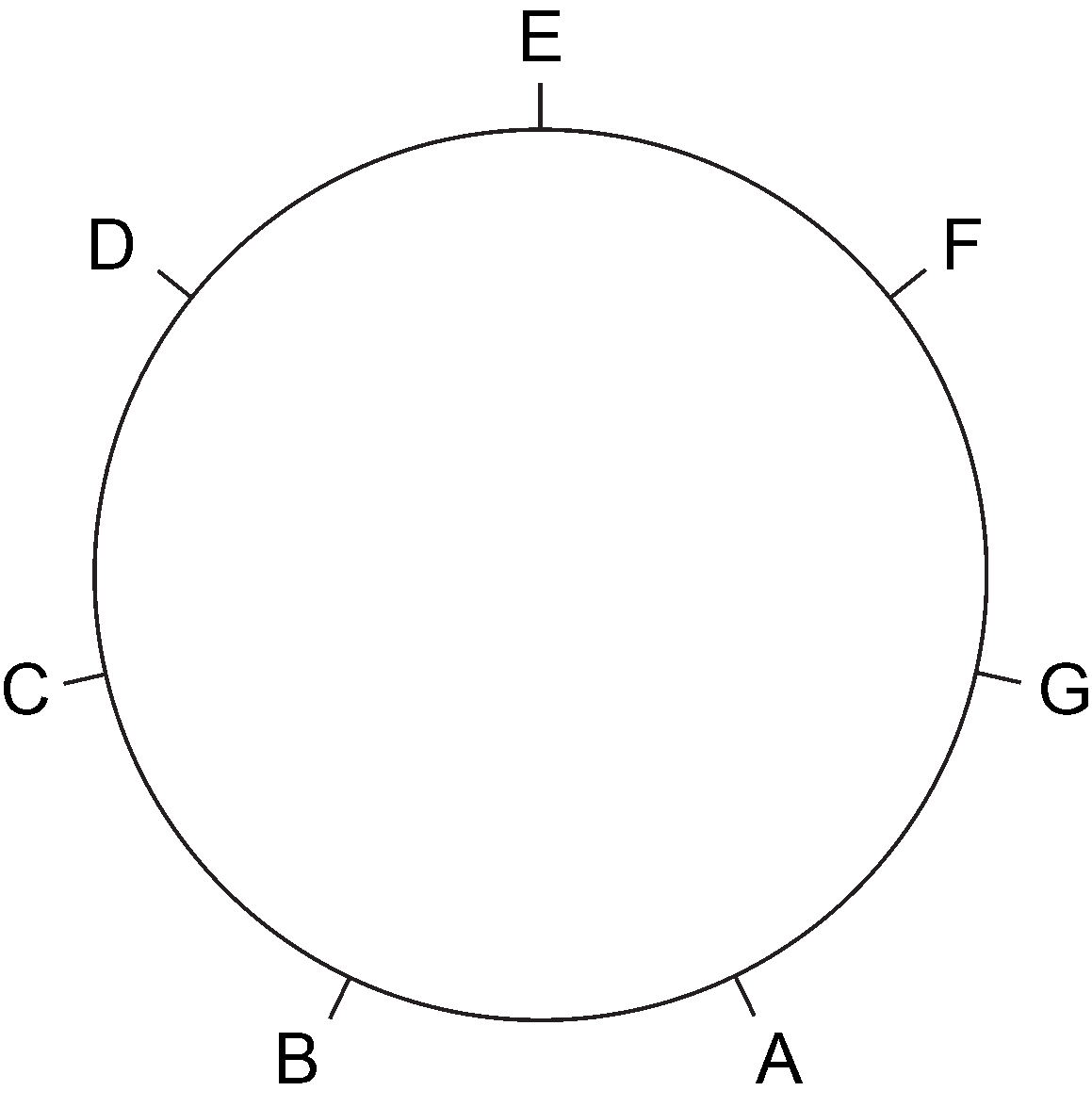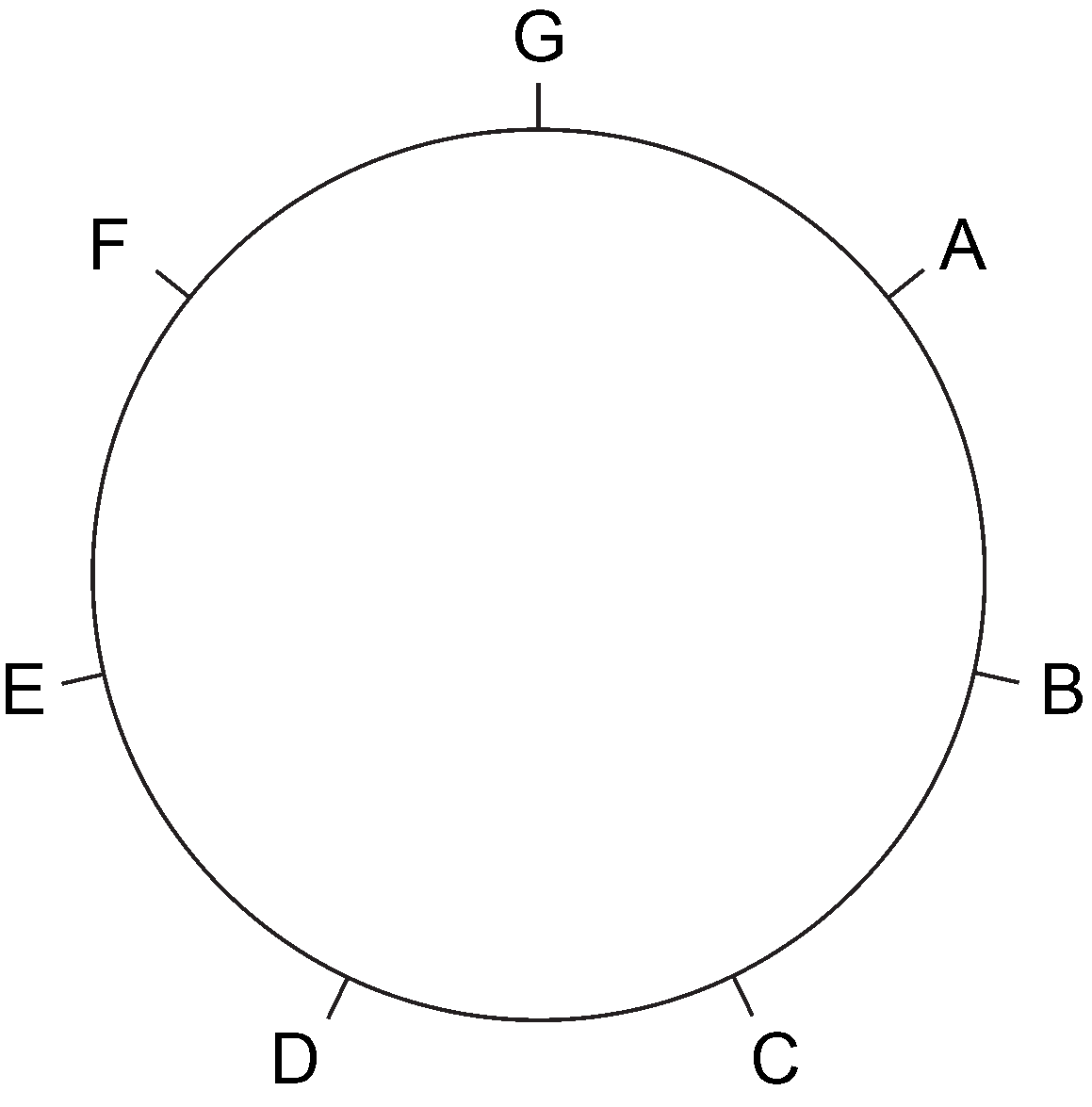
In the second representation above, it is possible to imaging rotating the circle in either direction, placing successive letter names at the top.  Completing this action seven times in either direction would represent a complete rotation through all pitch names.

Remember, finally, any of the seven letters from A to G can stand for a pitch (e.g. any given not D) or pitch class (e.g. all notes called D related by octave equivalence)

The piano keyboard—”White” notes

An important and practical way to visualise and understand notes and their recurring patterns of letter names is through the piano keyboard, with its familiar pattern of “white” and “black” keys. Each key represents a note.

In the segment of the keyboard shown above, there are two complete rotations through all of the pitch names (and further incomplete rotations to the left and right).  As you can see, reading from left to right, adjacent white notes read up from A to G and then start again (just like the line or circle of letter names in the previous section.)

At this stage, we are only naming the “white” keys—the “black” keys also have names, but these names are dependent on the white keys adjacent to them (we will get to this later).  However, the as-yet unnamed black keys are important, because they reveal a pattern that identifies where the rotation through the letters A to G recurs.  The black keys group into bunches of two and three, in a regularly recurring pattern (shown above the keyboard).  Going from left to right (which in pitch terms is from lower to higher), you will notice that A is always between the second and third in the group of three black keys.  All the other notes (B to G) also have their own unique position relative to the groups of black keys.

If we count (or play) each white key from one letter to the next instance of that same letter (or, complete one rotation through the letter names), we must use eight keys (including the ones we start and finish on).   Various rotations are shown below: A to A, D to D, C to C and again to the next C. The two over-lapping rotations from C to C and to the next C, shows that we have to restart the count at “1” again for a subsequent rotation.

This means that the “distance” measured in terms of the number of keys (or notes) needed to get from one instance of the same letter to the next is eight and this distance, or interval (to use the correct music theory term), is therefore called an octave (from the Latin octavus, meaning “eighth”).

Notes that are an octave apart always share the same letter name, and always have a fundamental frequency ratio of 1:2, or 2:1 (depending whether the first note is lower or higher).^[5]

If we now go to a complete piano keyboard (88 keys in all), we can see the more-or-less standard range of notes that is used in Western music.

If we wish to distinguish specific notes as opposed to talking about pitch class, a system of numbering can be used, called Scientific Pitch Notation, which gives each note both a letter name and a (usually subscript) number, starting at “0” for the lowest pitch.  In the illustration above, there are several things to note.

The most important of these is that the numbering of pitch names references rotations starting on C, not on A.  In other words, our reference point is C.  This may seem counterintuitive, but it is a standard convention.

C4 is called “middle C”—it sits roughly in the middle of the total range of piano keys.  The first A above it (A4) is the tuning standard A440 (introduced earlier).

The various groups of notes with the same number are understood to belong to a specific range (sometimes also called an octave, slightly misleadingly). These have conventional designations, some of which also relate to the length of organ pipes. These are shown underneath the horizontal brackets.

Scientific Pitch Notation is not the only way of identifying specific pitch. There are a number of other systems, probably the most well-known of which is the Helmholtz system (shown above the keyboard).  It also takes C as a reference.

Finally, for reference, the illustrations also shows the conventional ranges for the standard human voice types (bass, tenor, alto and soprano).